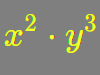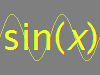5.4.1 Arithmetic Properties 🔧
Constant Rule
$$\frac{d}{dx}(C)=0$$
Constant Multiple Rule
$$\frac{d}{dx}(C·f(x))=C·\frac{d}{dx}f(x)$$
Sum & Difference Rules
$$\frac{d}{dx}\big(f(x)\pm g(x)\big)=f'(x)\pm g'(x)$$
5.4.2 Product Rule 🔧
$$\frac{d}{dx}\big(f(x)·g(x)\big)=f'(x)·g(x)+f(x)·g'(x)$$
Proof
5.4.3 Quotient Rule 🔧
$$\frac{d}{dx}\bigg(\frac{f(x)}{g(x)}\bigg)=\frac{g(x)·f'(x)-f(x)·g'(x)}{g(x)^2}$$
Proof
5.4.4 Power Rule 🔧
$$\frac{d}{dx}x^n=n·x^{n-1},\medspace \forall x\isin\R$$
Proof
5.4.5 Mean Value Theorem for Derivatives 🔧
$$f'(c)=\frac{f(b)-f(a)}{b-a}$$

5.4.6 Implicit Differentiation (Chain Rule) 🔧
Proof
5.4.7 Derivative of an Inverse Function 🔧
Proof
5.4.8 L'Hôpital's Rule 🔧
The indeterminate form 0·∞
The indeterminate form ∞–∞
Exponential Indeterminate Forms
Proof
5.4.9 Derivative of $e$ Exponents 🔧
$$\frac{d}{dx}e^x=e^x$$
Proof
5.4.A Derivative of Logarithmic Functions 🔧
Proof
5.4.B Derivative of Exponential Functions 🔧
$$\frac{d}{dx}b^x=b^x·\ln(b)$$
Proof
5.4.C Derivative of a Variable Raised to Itself 🔧
$$\frac{d}{dx}x^x=x^x·\big(1+\ln(x)\big)$$
Proof