5.7.1 Unit Hyperbola Definitions 🔧
Hyperbolic trig functions operate similarly as the trig functions on the unit circle, except they neither rotate nor have periodicity.

$$\sinh(\theta)=y/h\qquad\text{csch}(\theta)=h/y$$
$$\cosh(\theta)=x/h\qquad\text{sech}(\theta)=h/x$$
$$\tanh(\theta)=y/x\qquad\coth(\theta)=x/y$$
$$\theta=\sinh^{-1}(y/h)\qquad\theta=\text{csch}^{-1}(h/y)$$
$$\theta=\cosh^{-1}(x/h)\qquad\theta=\text{sech}^{-1}(h/x)$$
$$\theta=\tanh^{-1}(y/x)\qquad\theta=\coth^{-1}(x/y)$$
Relations
A unit circle rotated one half radian on the unit double cone along its tangential axis results in the unit hyperbola.

The number $1$ rotated one half radian in the complex plane results the imaginary unit $i$.

The insertion of $i$ into the unit circle equation results in the unit hyperbola equation.
$$x^2+(i·y)^2=1\medspace →\medspace x^2-y^2=1$$

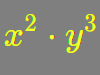





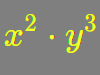

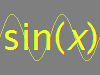




 $$\sinh(\theta)=y/h\qquad\text{csch}(\theta)=h/y$$
$$\cosh(\theta)=x/h\qquad\text{sech}(\theta)=h/x$$
$$\tanh(\theta)=y/x\qquad\coth(\theta)=x/y$$
$$\theta=\sinh^{-1}(y/h)\qquad\theta=\text{csch}^{-1}(h/y)$$
$$\theta=\cosh^{-1}(x/h)\qquad\theta=\text{sech}^{-1}(h/x)$$
$$\theta=\tanh^{-1}(y/x)\qquad\theta=\coth^{-1}(x/y)$$
$$\sinh(\theta)=y/h\qquad\text{csch}(\theta)=h/y$$
$$\cosh(\theta)=x/h\qquad\text{sech}(\theta)=h/x$$
$$\tanh(\theta)=y/x\qquad\coth(\theta)=x/y$$
$$\theta=\sinh^{-1}(y/h)\qquad\theta=\text{csch}^{-1}(h/y)$$
$$\theta=\cosh^{-1}(x/h)\qquad\theta=\text{sech}^{-1}(h/x)$$
$$\theta=\tanh^{-1}(y/x)\qquad\theta=\coth^{-1}(x/y)$$
 The number $1$ rotated one half radian in the complex plane results the imaginary unit $i$.
The number $1$ rotated one half radian in the complex plane results the imaginary unit $i$.
 The insertion of $i$ into the unit circle equation results in the unit hyperbola equation.
$$x^2+(i·y)^2=1\medspace →\medspace x^2-y^2=1$$
The insertion of $i$ into the unit circle equation results in the unit hyperbola equation.
$$x^2+(i·y)^2=1\medspace →\medspace x^2-y^2=1$$